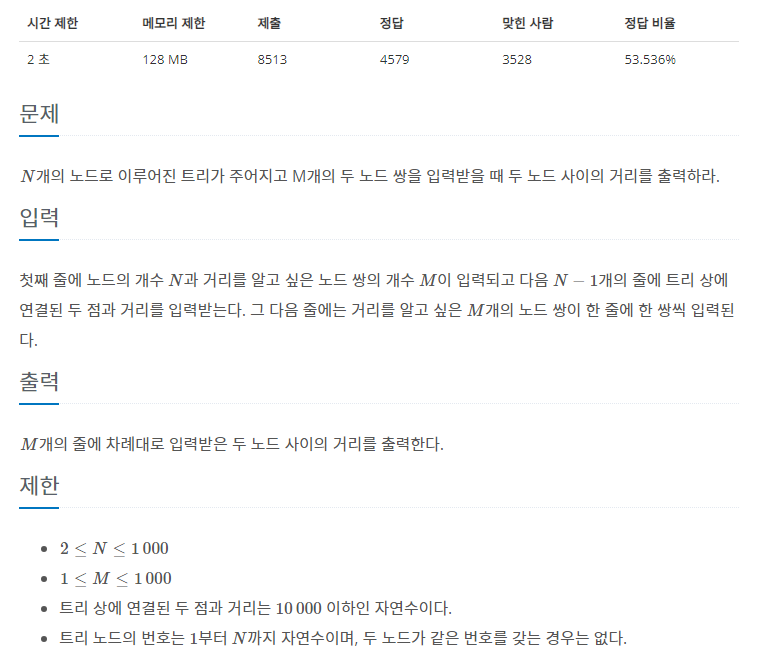
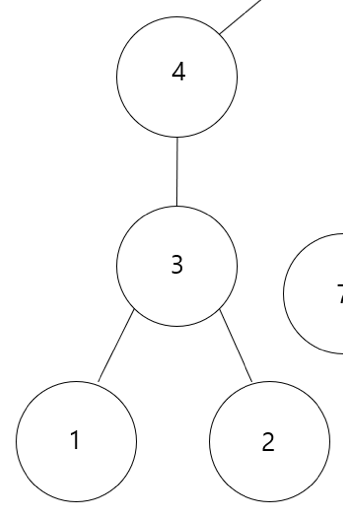
문제
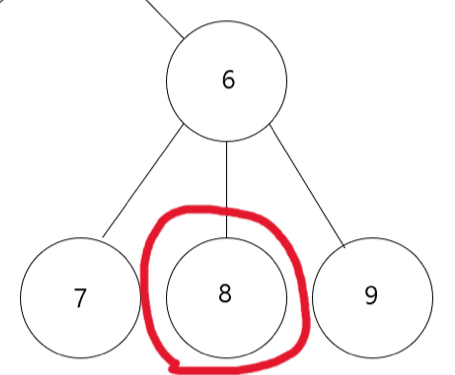
접근 방식
내리 칭찬이 있어서 부하는 상사가 받은 칭찬 + 상사한테 받을 칭찬을 받을 수 있다.
트리에서 상사는 부모고 부하는 자식이니 루트부터 방문을 하면서
부하에게 현재까지 본인이 받은 칭찬을 넘겨주기만 하면 된다.
조심해야할게 한 명의 부하가 여러번 칭찬을 받을 수 있어서
입력을 받을 때
부하 번호 = 부하가 직속 상사로부터 받은 칭찬 이 아니라
부하 번호 += 부하가 직속 상사로부터 받은 칭찬 이 되어야 한다.
풀이
public class Main {
private static final ArrayList<ArrayList<Integer>> near = new ArrayList<>();
private static int[] compliments;
public static void main(String[] args) throws IOException {
BufferedReader br = new BufferedReader(new InputStreamReader(System.in));
StringTokenizer st = new StringTokenizer(br.readLine());
StringBuilder sb = new StringBuilder();
int n = Integer.parseInt(st.nextToken());
int m = Integer.parseInt(st.nextToken());
int p;
compliments = new int[n + 1];
for (int i = 0; i <= n; i++) {
near.add(new ArrayList<>());
}
st = new StringTokenizer(br.readLine());
for (int i = 1; i <= n; i++) {
p = Integer.parseInt(st.nextToken());
if (p == -1) continue;
near.get(p).add(i);
}
while (m-- > 0) {
st = new StringTokenizer(br.readLine());
compliments[Integer.parseInt(st.nextToken())] += Integer.parseInt(st.nextToken());
}
dfs(1);
for (int i = 1; i <= n; i++) {
sb.append(compliments[i]).append(" ");
}
System.out.println(sb);
}
private static void dfs(int cur) {
for (int next : near.get(cur)) {
compliments[next] += compliments[cur];
dfs(next);
}
}
}
'Java > Algorithms' 카테고리의 다른 글
| [백준] 2295번 : 세 수의 합 (1) | 2024.02.04 |
|---|---|
| [백준] 2250번 : 트리의 높이와 너비 (0) | 2024.01.29 |
| [백준] 20955번 : 민서의 응급 수술 (0) | 2024.01.28 |
| [백준] 1240번 : 노드사이의 거리 (0) | 2024.01.28 |
| [백준] 15681번 : 트리와 쿼리 (0) | 2024.01.28 |